Fractions: Teaching for Understanding

http://www.education.vic.gov.au/school/teachers/teachingresources/discipline/maths/continuum/Pages/fracdecint.aspx
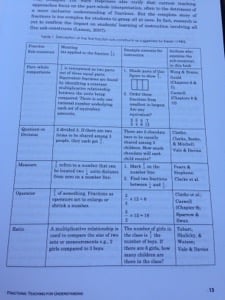
How did you work that out?
Use this to diagnose misconceptions/ current understanding.

Practical hints for the classroom teacher:
1. Give a greater emphasis to the meaning of fractions than on procedures for manipulating them.
2. Develop a general rule for explaining the numerator and denominator of a fraction. In the fraction a/b, b is the name or size of the part (e.g., fifths have this name because 5 equal parts can fill a whole) and a is the number of parts of that name or size
3. Emphasise that fractions are numbers, making extensive use of number lines in representing fractions and decimals.
4. Take opportunities early to focus on improper fractions and equivalences.
5. Provide a variety of models to represent fractions. Student made ones will help them to understand the importance of congruency when comparing fractional parts.
6. Link fractions to key benchmarks, and encourage estimation. Innovative strategy – residual thinking (when comparing 5/6 and 7/8 students may conclude that the first fraction requires 1/6 more to make the whole (“the residual”), while the second requires only 1/8 to make the whole (a smaller piece of number), so 7/8 is larger. Students sharing strategies is an important teaching strategy to encourage.
7. Give emphasis to fractions as division. Students need lots of opportunities to partition objects in sharing and other contexts to help build this notion.
8. Link fractions, decimals and percentages wherever possible. Students will choose to convert it to decimals or percentages, in order to make sense of it. This flexibility is to be encouraged, as percentages particularly seem to make sense to many students intuitively.
9. Take the opportunity to interview several students one-to-one to gain awareness of their thinking and strategies.
10. Look for examples and activities which can engage students in thinking about fractions in particular and rational number ideas in general. E.g., Cuisenaire rods:
What fraction of brown rod is the red rod?
If the purple is 2/3, which rod is the whole?
If the brown rod is 4/3, which rod is one?
If the blue rod is 1 1/2, which rod is 2/3?
Your brilliant question for another group!